Physicists find symmetry elemental in describing the physical world. "Fundamental symmetry principles dictate the basic laws of physics, control the stucture of matter, and define the fundamental forces in nature." says Leon M. Lederman, Nobel Laureate in physics. ("Symmetry and the Beautiful Universe", Lederman and Hill, 2004, Prometheus, N.Y.)
 |
How are we to understand this? How is the visual symmetry apparent all around us connected to the laws of physics? What is the link between the shape of objects and the laws of nature? That is what this exposition is about.
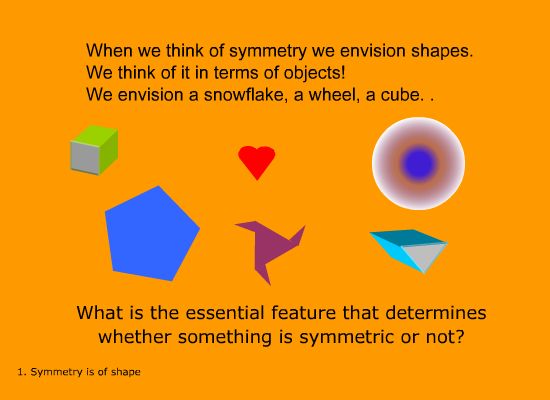
To begin we should have a clear idea of what visual symmetry is. We need an operational definition. Given any object how can we decide whether it has symmetry or not? What shall we do that will unerringly yield an unambiguous answer to the question, "Does this object have symmetry? Yes or no?"
What we must do is to inspect it. We scrutinize the object, looking at it from a multitude of perspectives. The test for symmetry is this: Does it look the same under two or more scrutinies? If it does then it has symmetry. If not it doesn't. The critical test for symmetry is therefore this: sameness under some altered scrutiny.
A name attaches to this particular symmetry. Every symmetry is nameable. The six altered scrutinies that produce the appearance of sameness form, by the rules of mathematics, what is called a group. The name of this group is C6, the cyclic group of order 6. And group theory is the mathematical generalization of visual symmetry. When Lederman says "symmetry principles dictate the basic laws of physics" he is saying that group theory - not the physical shape of things - dictates these laws.
Group theory describes visual symmetry quantitatively but it also contains abtract ideas of symmetry beyond shape. Remarkably, if we merely expand our concept of scrutiny to include taking measurements, then we can carry over the intuitive ideas about shape symmetry into the abstract realm of physics! Taking measurements is what one does to scrutinize nature. For example, the acceleration measurement of a falling object produces the same number at a later time as at the earlier. An altered scrutiny in time produces sameness. There is thus a symmetry idea associated with this finding in nature. And it has nothing to do with shape. In physics the formal term for sameness under altered scrutiny is invariance.
Based on this elemental notion of what constitutes symmetry - sameness under altered scrutiny - the whole structure of symmetry in physics can be worked out mathematically. Click here to see how that is done. Because things are best explained using animated graphics, the detailed presentation is in motion graphic gallery format. (Select images, scroll text: independently)
Objective Reality
Group theory is the mathematical formulation of internal consistency in the description of things. We assume that the system being observed has an intrinsic character independent of the observer's perspective. It's there. It posseses an objective reality. On this assumption - that it's there - how the system is perceived under altered scrutiny must be a matter of logic. Its appearance follows the logic of objective reality; that just because we change our point of view in examining the system does not change the nature of the system. This figure illustrates the point.
Clicking the next button prepares us to see a transformation NOT due to altered scrutiny: something excluded in group theory. To see this click on play. This transformation (disappearance of the object) cannot be a result of the observer's altered scrutiny. Results not in accord with the intrinsic sameness of the system - with its objective reality - are excluded in Group Theory. The Theory is predicated on the elemental logic contained in the presumption of objective reality. This idea is elaborated in the detailed presentation.
Symmetry and Identity
The phrase - a perceived sameness under altered scrutiny - captures not only the essense of symmetry but also the notion of identity. When something is recognizably the same under many perspectives we grant it identity. An identification is made by labelling. The label tags what it is that we perceive as invariant. "If there were no invariants we could not define 'identity'" noted M.L.G. Redhead. (Redhead, M.L.G., "Symmetry in Intertheory Relations", (1975) Synthese, 32, 77-112) Group Theory has an innate taxonomic structure - a taxonomy for behavior. It assigns labels for behavior (appearance) under altered scrutiny. The irreducible representation labels of Group Theory are the identification markers - the labels of identity.
February 2015
Marvin Chester
email
© m chester 2015 Santa Cruz, CA